Fundamentals of Statistics contains material of various lectures and courses of H. Lohninger on statistics, data analysis and chemometrics......click here for more.

Home Statistical Tests ANOVA ANOVA and Regression
See also: regression, ANOVA 

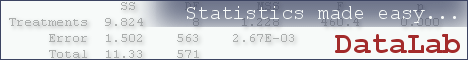
ANOVA and Regression

A powerful procedure to obtain more information on the quality of a regression model is the analysis of variances (ANOVA). The idea behind ANOVA is to split the variances within a model into several parts, which then can be set in relationship to each other, thus uncovering facts about the model. ANOVA can thus be used to check the validity a model, and the goodness (or, lack) of fit. Basically we have to distinguish between two cases:
 

  • analysis of variances without replicate measurements, and
  • analysis of variances including replicate measurements.


The second case is the more powerful and allows the collection of further information on the model involved.

The general scheme of the breakdown of errors is as follows
 



In the case of measurements without replicates the ANOVA has to be carried out according to the following scheme. If the resulting F value exceeds the critical value Fα;(1,n-2), the null hypothesis H0 that the slope b of the line is equal to zero has to be rejected.

 
Home Statistical Tests ANOVA ANOVA and Regression