Grubbs' Outlier Test

Fundamentals of Statistics contains material of various lectures and courses of H. Lohninger on statistics, data analysis and chemometrics......click here for more.

Home

Statistical Tests

Outlier Tests

Index

Grubbs' Outlier Test

Grubbs' outlier test (Grubbs 1969

and Stefansky 1972

) checks normally distributed data for outliers. This implies that one has to check whether the data show a normal distribution before applying the Grubbs test. The Grubbs test always checks the value which shows the largest absolute deviation from the mean. If an outlier has been identified and removed, the test must not be repeated without adapting the critical value.

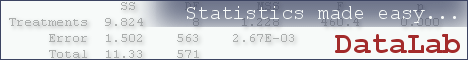
The application of the test is quite simple and straightforward: one searches the maximum of the absolute differences between the values x_i and the mean . The result is divided by the standard deviation of the sample. If the resulting test statistic g is greater than the critical value, the corresponding value can be regarded to be an outlier. An extract of the critical values is shown in the following table:

n g_crit
α=0.05 g_crit
α=0.01 n g_crit
α=0.05 g_crit
α=0.01 n g_crit
α=0.05 g_crit
α=0.01

3 1.1543 1.1547 15 2.5483 2.8061 80 3.3061 3.6729

4 1.4812 1.4962 16 2.5857 2.8521 90 3.3477 3.7163

5 1.7150 1.7637 17 2.6200 2.8940 100 3.3841 3.7540

6 1.8871 1.9728 18 2.6516 2.9325 120 3.4451 3.8167

7 2.0200 2.1391 19 2.6809 2.9680 140 3.4951 3.8673

8 2.1266 2.2744 20 2.7082 3.0008 160 3.5373 3.9097

9 2.2150 2.3868 25 2.8217 3.1353 180 3.5736 3.9460

10 2.2900 2.4821 30 2.9085 3.2361 200 3.6055 3.9777

11 2.3547 2.5641 40 3.0361 3.3807 300 3.7236 4.0935

12 2.4116 2.6357 50 3.1282 3.4825 400 3.8032 4.1707

13 2.4620 2.6990 60 3.1997 3.5599 500 3.8631 4.2283

14 2.5073 2.7554 70 3.2576 3.6217 600 3.9109 4.2740

There is a one-sided alternative which allows to test either the minimum x_min or the maximum x_max of the entire data set. The test statistics calculates according to the following formulas:

A value can be regarded an outlier if the statistic g is greater than the critical value. Please note that in the case of the one-sided test the critical values are different. An extract is given below:

n g_crit
α=0.05 g_crit
α=0.01 n g_crit
α=0.05 g_crit
α=0.01 n g_crit
α=0.05 g_crit
α=0.01

3 1.1531 1.1546 15 2.4090 2.7049 80 3.1319 3.5208

4 1.4625 1.4925 16 2.4433 2.7470 90 3.1733 3.5632

5 1.6714 1.7489 17 2.4748 2.7854 100 3.2095 3.6002

6 1.8221 1.9442 18 2.5040 2.8208 120 3.2706 3.6619

7 1.9381 2.0973 19 2.5312 2.8535 140 3.3208 3.7121

8 2.0317 2.2208 20 2.5566 2.8838 160 3.3633 3.7542

9 2.1096 2.3231 25 2.6629 3.0086 180 3.4001 3.7904

10 2.1761 2.4097 30 2.7451 3.1029 200 3.4324 3.8220

11 2.2339 2.4843 40 2.8675 3.2395 300 3.5525 3.9385

12 2.2850 2.5494 50 2.9570 3.3366 400 3.6339 4.0166

13 2.3305 2.6070 60 3.0269 3.4111 500 3.6952 4.0749

14 2.3717 2.6585 70 3.0839 3.4710 600 3.7442 4.1214

Home

Statistical Tests

Outlier Tests

Grubbs' Outlier Test